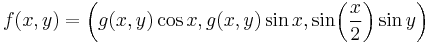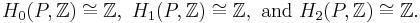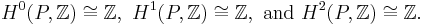Pinched torus

In mathematics, and especially topology and differential geometry, a pinched torus (or croissant surface) is a kind of two-dimensional surface. It gets its name from its resemblance to a torus that has been pinched at a single point. A pinched torus is an example of an orientable, compact 2-dimensional pseudomanifold.^[1]

1 Parametrisation
2 Topology
- 2.1 Homology
- 2.2 Cohomology
3 References

Parametrisation

A pinched torus is easily parametrisable. Let us write g(x,y) = 2 + sin(x/2).cos(y). An example of such a parametrisation − which was used to plot the picture − is given by ƒ : [0,2π)² → R³ where:

$f(x,y) = \left( g(x,y)\cos x , g(x,y)\sin x , \sin\!\left(\frac{x}{2}\right)\sin y \right)$

Topology

Topologically, the pinched torus is homotopy equivalent to the wedge of a sphere and a circle.^[2]^[3] It is homeomorphic to a sphere with two distinct points being identified.^[2]^[3]

Homology

Let P denote the pinched torus. The homology groups of P over the integers can be calculated. They are given by:

$H_0(P,\Z) \cong \Z, \ H_1(P,\Z) \cong \Z, \ \text{and} \ H_2(P,\Z) \cong \Z.$

Cohomology

The cohomology groups of P over the integers can be calculated. They are given by:

$H^0(P,\Z) \cong \Z, \ H^1(P,\Z) \cong \Z, \ \text{and} \ H^2(P,\Z) \cong \Z.$

References

^ Brasselet, J. P. (1996). "Intersection of Algebraic Cycles". Journal of Mathematical Sciences (Springer New York) 82 (5): 3625 − 3632. http://www.springerlink.com/content/ju28j2wqm174hx10.
^ ^a ^b Hatcher, Allen (2001), Algebraic Topology, Cambridge University Press, ISBN 0521795400
^ ^a ^b Allen Hatcher. "Chapter 0: Algebraic Topology". http://www.math.cornell.edu/~hatcher/AT/ATch0.pdf. Retrieved August 6, 2010.